MACRO-interval

Let us begin the sequential analysis of structural laws of systems construction from the middle scale interval - from the interval where electromagnetism dominates in the construction of objects of nature by 20 orders of magnitude.

The macro interval begins at sizes \(10^{-13}\) cm. On this scale, the most significant systems are atomic nuclei (CLASS #4).

Recall that, purely for reasons of convenience, the three intervals of 20 orders of magnitude each were named: the middle interval - Macro-, the left - Micro-, the right - Mega-. Further we will consider them mainly in integer values.

They have predominantly the so-called polycentric structure (see Fig. 1.22)1.

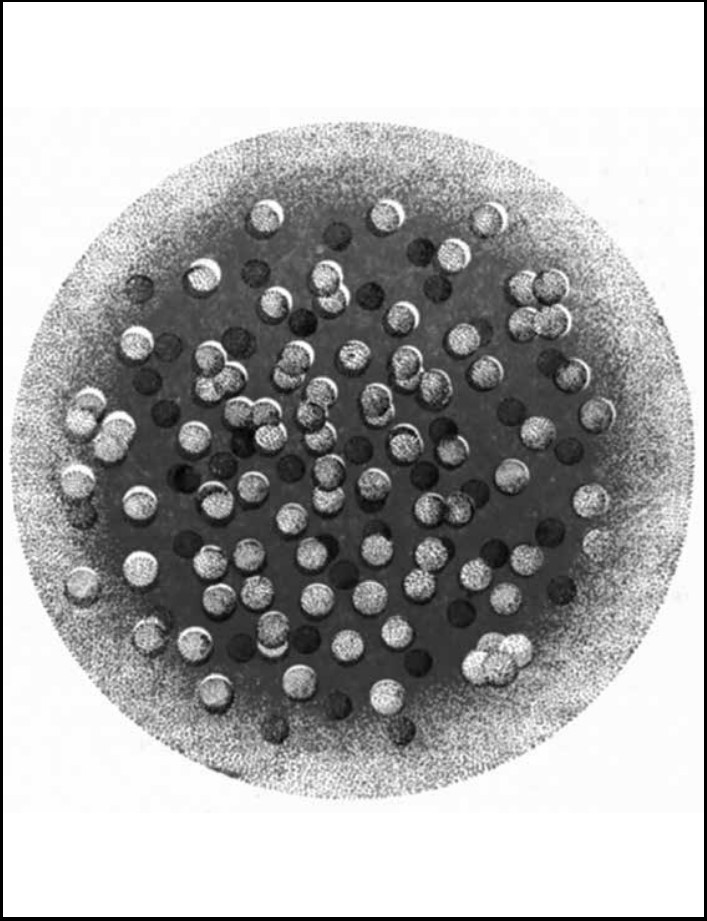
Fig. 1.22. Model of the spherical nucleus of the gold atom. The nucleus has a typical internal polycentric structure and consists of 197 nucleons: protons (light clumps) and neutrons (dark clumps). There is a lot of empty space in the nucleus, as the distance between the centers of the nucleons is about 2 fermi, which is noticeably larger than two nucleon radii. The density of nucleons and the intensity of powerful nuclear forces (thick color) are uniform near the center of the nucleus and gradually fall toward its surface (pale color)

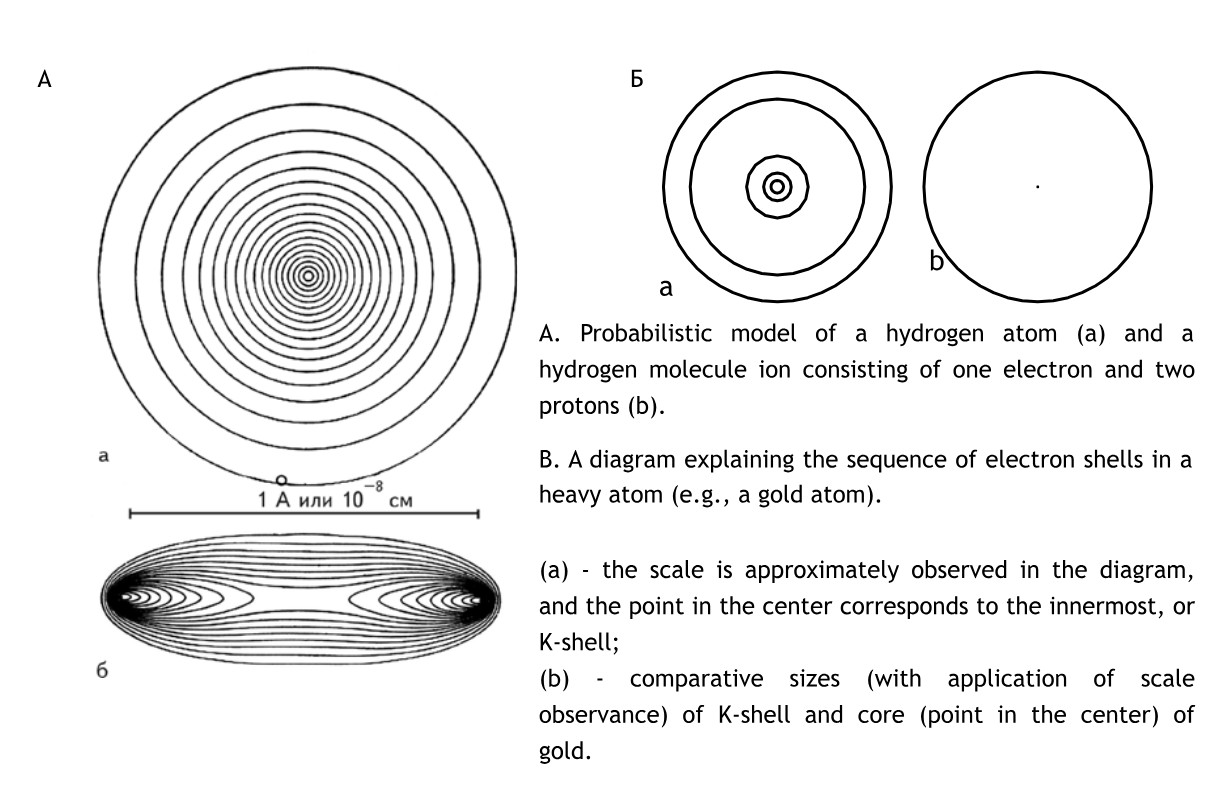Fig. 1.23. Figures A and B show the clearly monocentric structure of the atom: nucleus, shells, and spherical shape. (From the article "Particles and Forces" by R. Peierls. In the book: Fundamental Structure of Matter. M.: Mir, 1984).

This is the same "Thompson's pudding", rejected in its time for the structure of atoms, only \(10^{5}\) times smaller in size, and referring exclusively to the positively charged and neutral particles of the atomic nucleus - nucleons. The sizes of nuclei do not exceed \(10^{-12}\) cm, then, as we have already written, in nature there is an unexplained failure of structures down to the size of mesoatoms - \(10^{-10}\) cm. However, the structure of the atoms themselves, in contrast to the structure of their nuclei, is already fundamentally different - predominantly monocentric (see Fig. 1.23)2.

Thus, if we follow along the S-axis the evolution of matter at the early stages of its formation in the nascent Universe from nuclear scales to atomic scales, we find a certain qualitative jump in the types of systems. Up to a certain threshold of scales the structures are polycentric and have no distinguished nucleus. After the threshold there appear completely different structures - atoms with a pronounced nucleus.

In an atom, it is the nucleus that determines the fundamental properties and has orders of magnitude more mass and binding energy. Such a sharp material separation of the center is a unique phenomenon for nature, especially since the size of the nucleus is negligibly small in relation to the whole atom.

If we transfer the proportions of the atom to the social scale, a person occupying the position of the nucleus will be 200 kilometers away from another "nucleus man". However, at the same time, each of them will control the situation on a territory of more than 40,000 square kilometers!

The monocentric structure of the atom has an additional feature - the shell structure, because electrons are "spread" on their shells with fixed diameters. Thus, the structure of the atom differs from the structure of the nucleus in that it contains a functionally important nucleus and an internal shell structure.

In principle, it can be argued that the entire S-axis region from nuclei (\(10^{-13}\) cm) to atoms (\(10^{-8}\) cm) is occupied by monocentric structures, and as one moves from proton to hydrogen atom, the degree of monocentricity of the structures increases (from No. 8 to No. 1, see Fig. 1.21). However, the section of the S-axis dominated by monocentric systems occupies a very short interval, about 5 orders of magnitude. The dominance of monocentric structures almost ends after the atomic scale range. If we move a little to the right along the S-axis, we immediately enter the region of polycentricity dominance (see Fig. 1.24)3.

So, let us note an important phenomenological fact: on a short scale interval - from atomic nuclei to atoms themselves - the structure of matter makes a fundamental jump from one pole to another: from polycentrism to monocentrism.

Let us go back and consider in more detail the nature of polycentrism of atomic nuclei.

The nucleus of helium or other elements of the first numbers of the TEM (Mendeleev's table of elements) is lumpy rather than spherical.

Only a few nuclei have the shape of a sphere, namely those with the number of nucleons close to the magic numbers known in nuclear physics - 8, 20, 28, 50, 82, 126.

Most nuclei, on the other hand, have either a "hard" deformed" shape or a "soft" shape that is constantly changing.

Apparently, nonspherical nuclei can be divided into two categories.....

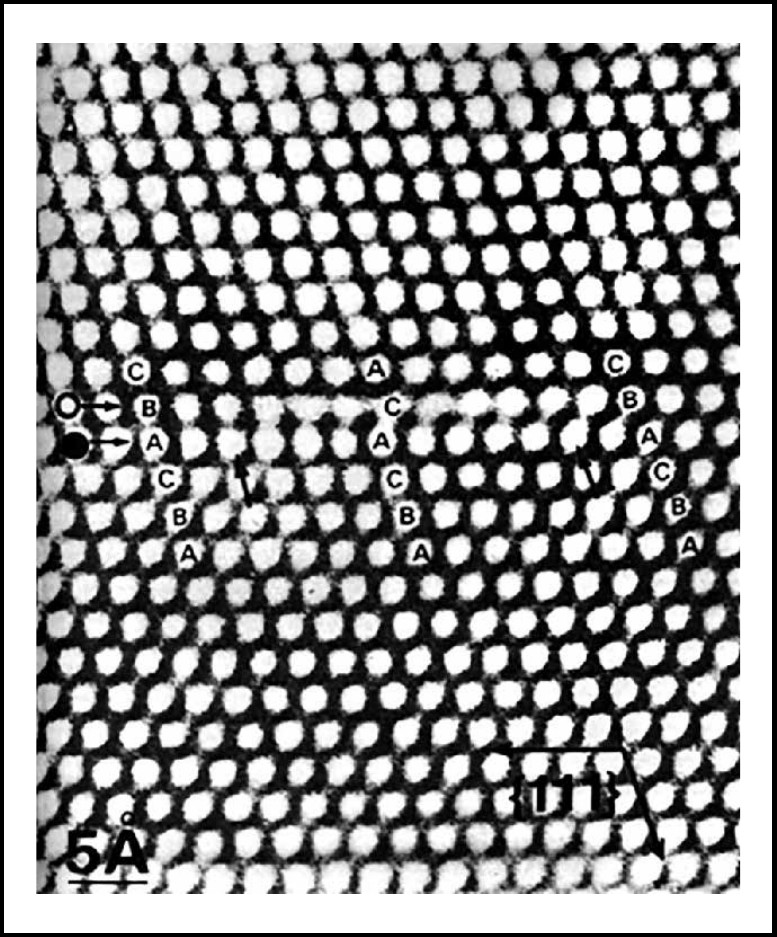
Fig. 1.24: The crystal lattice of gold. Photograph taken with an electron microscope. Each white dot is a gold atom located in the crystallographic plane

The first category includes "rigidly deformed" kernels. These nuclei mostly have a stable cigar shape. They are elongated spheroids with one long and two short axes. The other category consists of 'soft' ones, whose shape is highly variable ... mainly a variety of asymmetric ellipsoids ... as well as some spherical and oblong structures and flattened spheroids ... "2

Thus, we see that the shape of atomic nuclei only for some elements acquires a spherical form, and in general there are even elongated (linear) structures among them, and almost flat (two-dimensional). However, all of them have rotational symmetry axes. As for the central core in the nucleus, it simply does not exist. In other words, "the nucleus has no core "4 The density of charges and mass does not increase toward the center (see Fig. 1.22). Inner shells are found only in spherical nuclei, in them "the charge density does not decrease with increasing distance from the center, but fluctuates."5 Therefore, in atomic nucleus physics two alternative models are successfully used in different cases: drop and shell models.3.

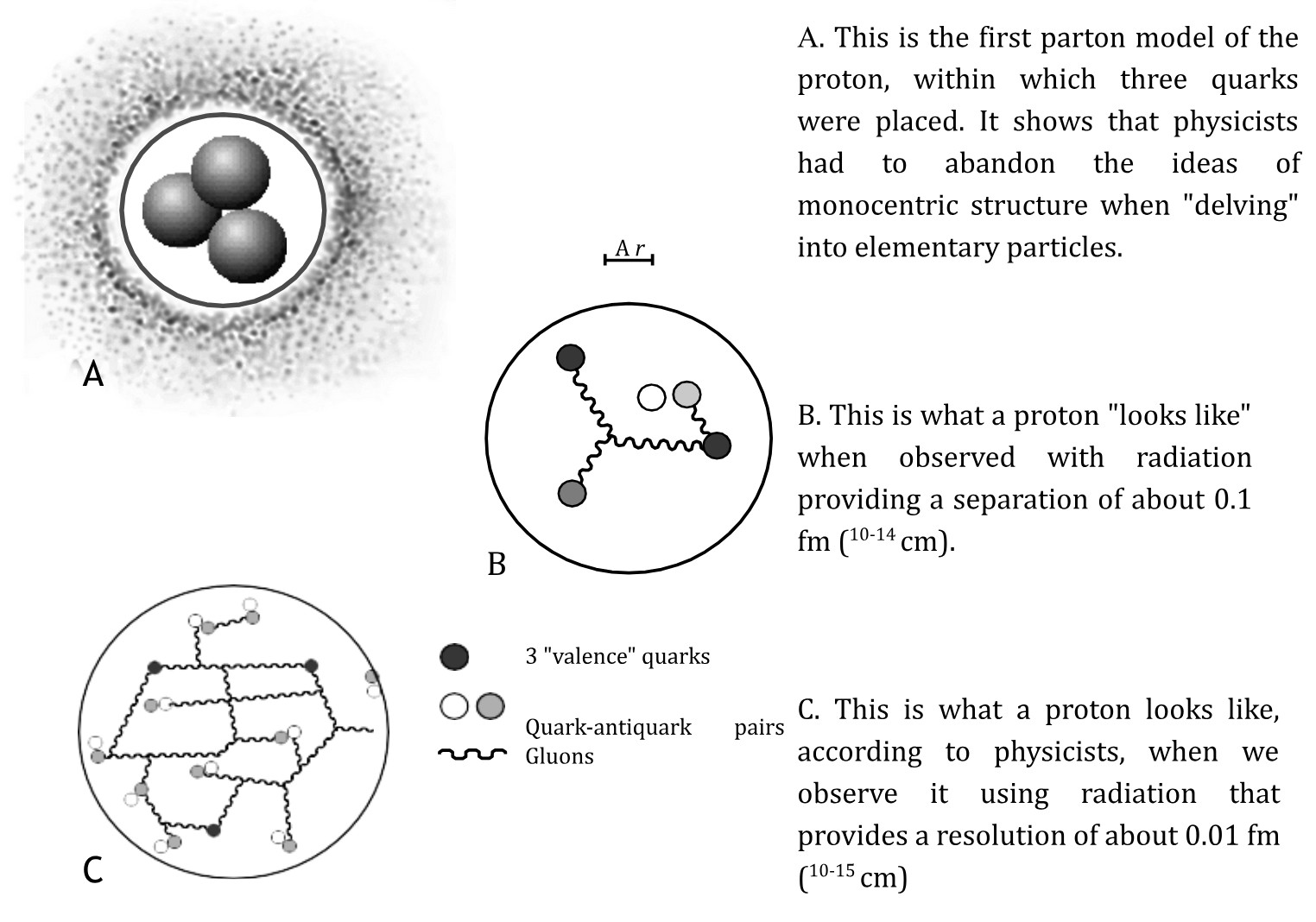
Fig. 1.25. These models show that the degree of polycentricity can increase as the proton penetrates deeper into the proton. However, on a scale of ~\(10^{-13}\) cm, the spherical shape of the proton gives it one of the important features of monocentricity.

However, even large "atomic nuclei do not have a smooth surface; protons and neutrons are not evenly distributed over the surface, but tend to clump into alpha particles, making the surface 'lumpy.'"5 All this suggests that the polycentric type of structure dominates in the scale region of atomic nuclei, through which monocentric features only rarely "break through": spherical shape and shell-like internal structure of nuclei with the magic number of nucleons. In general, atomic nuclei should be attributed to the polycentric type of objects.

The simplest representative of nuclei is the hydrogen nucleus - the proton. Such nuclei in the Universe are the overwhelming majority, more than 70% of the mass of the Universe.

How is the PROTON organized? Does it have a central core, spherical shells? Its structure was presented to physicists as such for quite a long time. The inertia of the revolution made by Rutherford, who "beat" Thompson's polycentric model of the atom with the monocentric model, was the reason. The monocentric model of the proton was called the central core model. However, experimental data soon disproved this model. It was as if nature had mocked the inertia of the physicists' model thinking. It so stunned them that even the title of the paragraph on the structure of the nucleon in one of the classic books on nuclear physics slipped the appropriate mood: "The end of the core."6 The way out of the crisis was found by R. Feynman, who proposed the parton model (part - part - part), where the nucleon consists of parts, it has no shells and no core. "According to Feynman, the nucleon in its rest frame is a complex particle consisting of virtual point parts - partons."7

Obviously, such an internal structure of the nucleon is absolutely polycentric (see Fig. 1.25), although its shape is spherical, which is an external sign of monocentricity. Therefore, the proton can be referred to the MS-Dorm No. 5. So, although polycentrism is still widespread at distances from \(10^{-13}\) cm to \(10^{-12}\) cm, we can find in this range of scales some signs of monocentrism, although it is still very far from being complete. To the right of the \(10^{-12}\) cm point, polycentric structures are already practically impossible either to meet in nature or to form artificially (creation of hypernuclei is a big problem8).

We can say that polycentric structures, apparently dominant up to \(10^{-13}\) cm, manifested as early as in atomic nuclei, completely disappear beyond the threshold of \(10^{-12}\) cm.

Further, up to the size of \(10^{-8}\) cm, exclusively monocentric structures - atoms and their ions - are found in nature. This scale interval of total monocentrism (from \(10^{-10}\) cm to \(10^{-8}\) cm) is accompanied by all its three features: spherical shape, central nucleus and shell structure of electron orbits.

Thus, we note once again that the process of changing the type of structure from polycentric to monocentric occurs in the size range of \(10^{-13}\) cm ... \(10^{-12}\) cm, and at the boundary of \(10^{-8}\) cm atoms show us the classical monocentric structure. If we now trace the path of evolution of matter from atoms further along the S-axis (for inanimate matter), then at 15 orders of magnitude (from \(10^{-8}\) to \(10^{7}\) cm) we will meet mainly polycentric structures: molecules, crystals9, microcrystals, particles, grains, stones, meteorites, asteroids, etc., i.e. monocentric structures are practically absent (or extremely rare) in the macrocosm of inanimate nature.

Thus, we see that the Macro-interval of the S-axis is not equally populated by different types of structures; in general, the situation is as follows: monocentrism dominates at the first 5 orders, while polycentrism dominates at the remaining 15 orders (see Fig. 1.26). There are, however, very interesting exceptions.

In the world of inorganic matter, the return of monocentrism (though in a weak form, i.e. not by all signs) is observed exclusively in the size range of 10-100 microns, namely in the lowest point (in the trough) of the given fragment of SWS - the Macro-interval and, as it has already been noted, in the S-CENTER OF THE UNIVERSE (SCU).

For a better associative understanding of the problem, we will further use a new concept - POTENTIAL STABILITY TROUGH, which corresponds to the lower part of the CU between the humps. Then there will be only 7 potential stability troughs (5 full troughs and 2 half-troughs at the edges of the S-interval) on the model of the HU (see Chapter II).

We are talking about the discovered mysterious appearance of spherical shapes both among cosmic dust83 and in the dust of volcanic origin. The fact is that most dust of cosmic origin has an angular fragmentary shape, and meteorites of all sizes have a chaotic shape. In this long range from hundreds of angstroms to hundreds of kilometers, one never found any particular symmetry until one came across balls of cosmic origin (see Fig. 1.27A). It turned out that "the vast majority of background deposition balls are 20-60 μm in size "84 and almost all of them are between 10 and 100 μm in size. The number of balls deposited on Earth is conservatively estimated at \(10^{4}\) tons per year. The same balls have been found on the surface of the Moon. Balls of similar sizes have been found in volcanic dust, although the conditions of their formation are very far from the conditions of formation of space balls. So, nature creates spherical forms in the size range from 10 to 100 microns regardless of the conditions of formation and almost independently of the chemical composition. This is especially surprising against the background of angular and typically polycentric structures for all crystalline matter in the range of 15 orders of the Macro-interval.

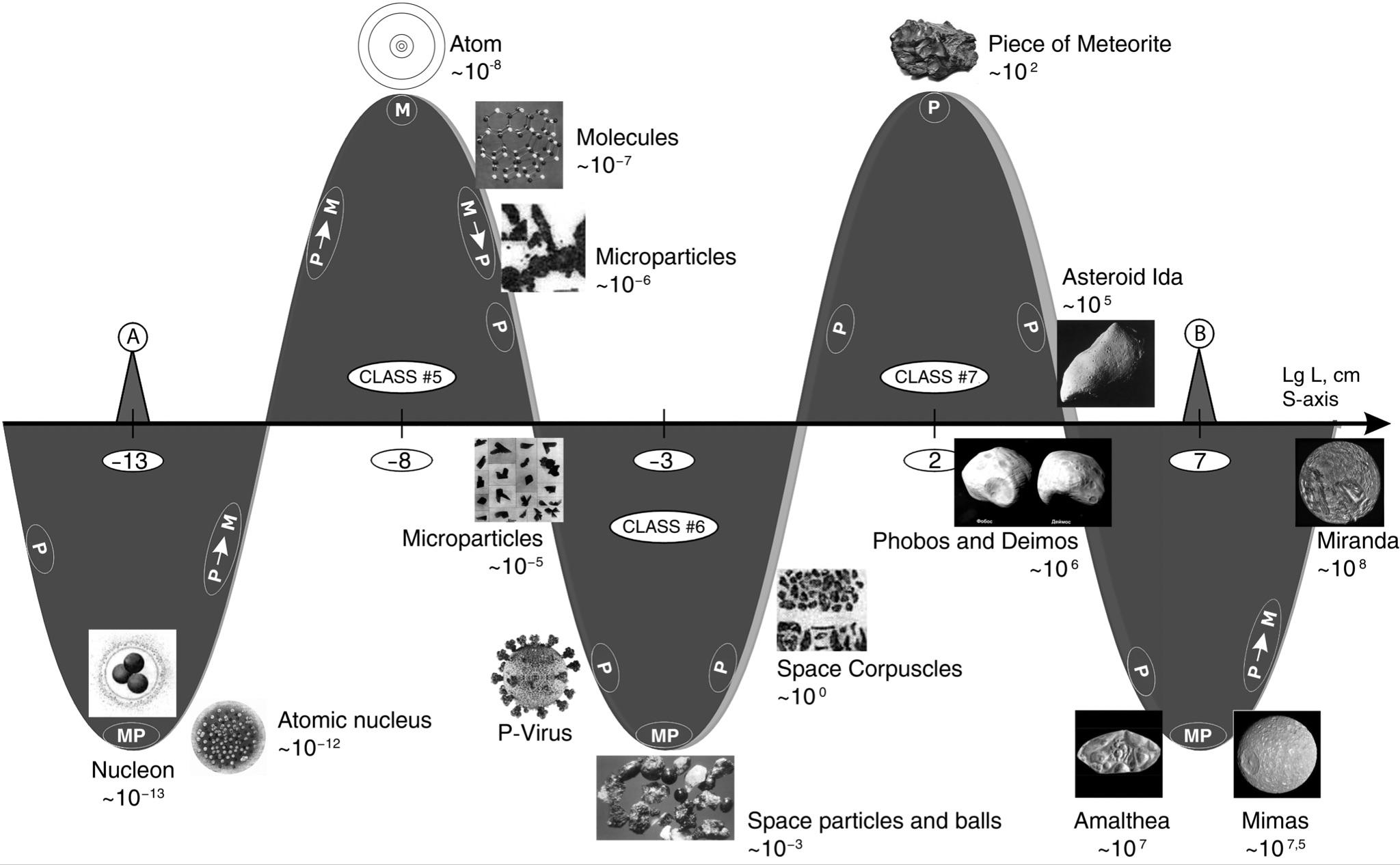Fig. 1.26. Monocentrism and polycentrism at 20 orders of the Macro interval (from A to B electromagnetic interactions dominate). Dimensions are given in cm

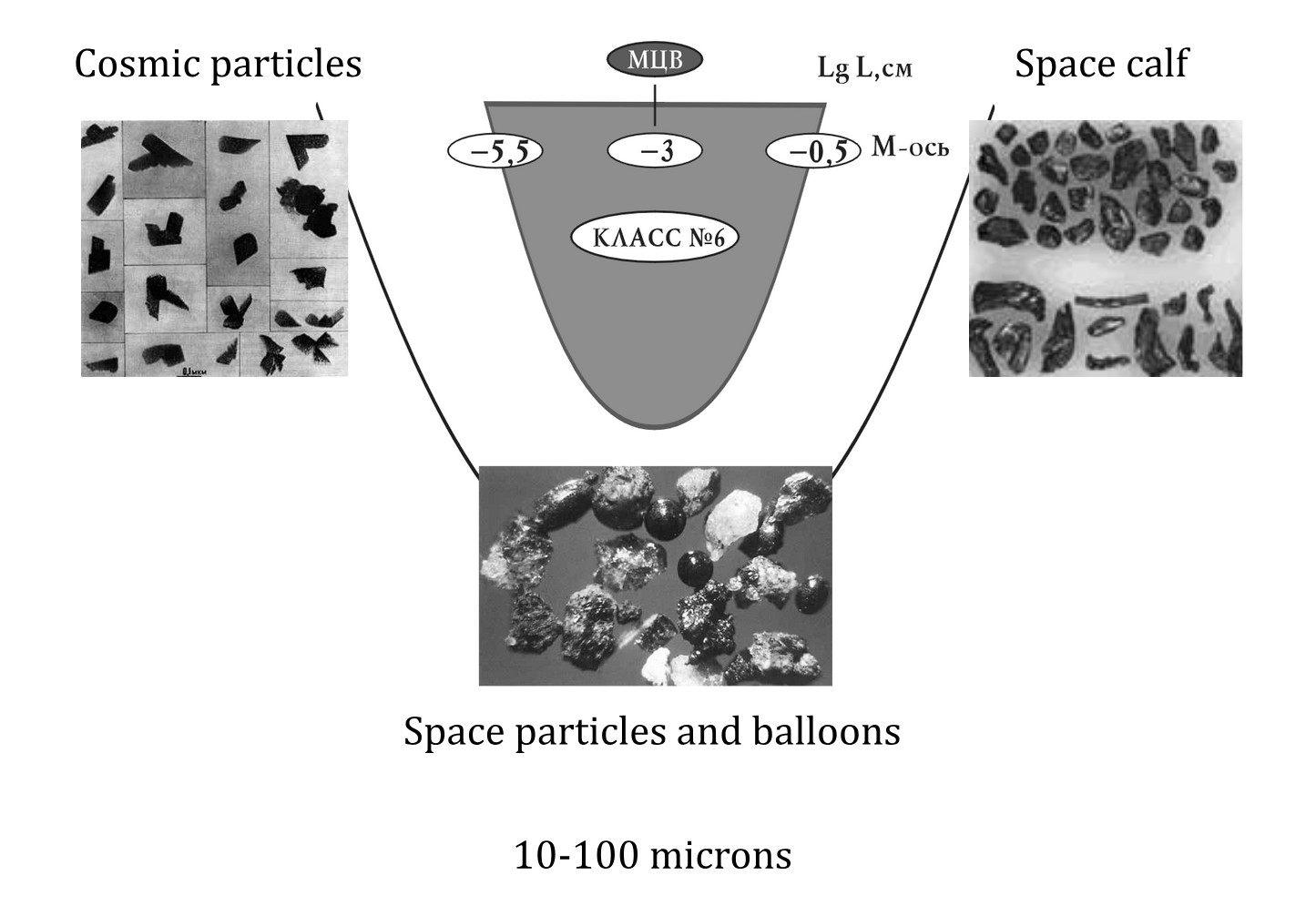Fig. 1.27A. For reasons still unclear to science, cosmic and volcanic dust particles in the size range from 10 to 100 μm often acquire a spherical perfect shape, although their "neighbors" in size, both to the left and to the right, never occur in the same spherical shape

Fig. 1.27B. As one moves along the S-axis from left to right in the "nuclear" class of the life wave, the symmetry of biosystems increases from the simplest types to the limiting ones (spherical and radial) in the region of the ICV (10...100 μm). Then, after passing the zone of ICV, it gradually decreases until it reaches the simplest form - mirror symmetry in the size region for animals, fish, birds, etc.

We failed to find any mention of nuclei and shells in marbles. It can be assumed that in some cases other features of monocentrism may appear in balls, but it is unlikely to expect the presence of all three features in their structure.

Thus, we note that the rare manifestation of monocentrism in the macrocosm coincides with the presence in this range of sizes of the stability trough on the SW, and, moreover, its exact coincidence with the scale center of the Universe. Obviously, the unique appearance of the spherical shape of balls in this range of scales is related to these factors, because it is known: the more symmetric the shape, the more stable the object.

BIOSYSTEMS (CLASSES #6, #7, #8). If we consider the S-interval for biosystems, at its beginning we have the same "primary bricks" as for non-living systems - atoms. Then the "fork" begins.

In non-living systems atoms and molecules form crystalline, amorphous and other simplest systems dominated by the principles of repetition symmetry that form ordered and homogeneous structures. The complication of structures for non-living systems is suspended at this stage.

For biosystems, the complication of structures continues further almost without interruption. Biomolecules evolve into protein molecules, followed by viruses, protozoa, plants and multicellular animals.

However, like a large-scale series of non-living systems, a number of protein systems (except for cells, which occupy a rather narrow interval of the S-axis) are represented mainly by P-structures (see Fig. 1.27B). Let us take a closer look at perhaps the only exception to this series of P-centered structures, the eukaryotic cell (a cell with a nucleus) (see Fig. 1.28). As already mentioned, the average size of eukaryotic cells of almost all species is in the range from 10 to 100 µm.

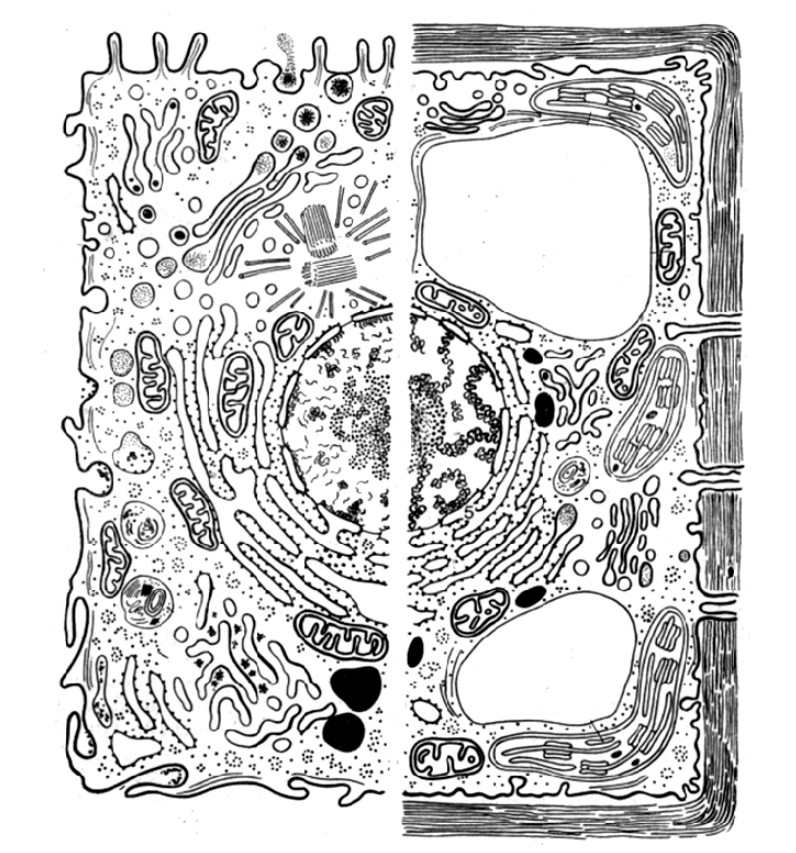
Fig. 1.28. MP structure of a eukaryotic cell (there is a central nucleus)

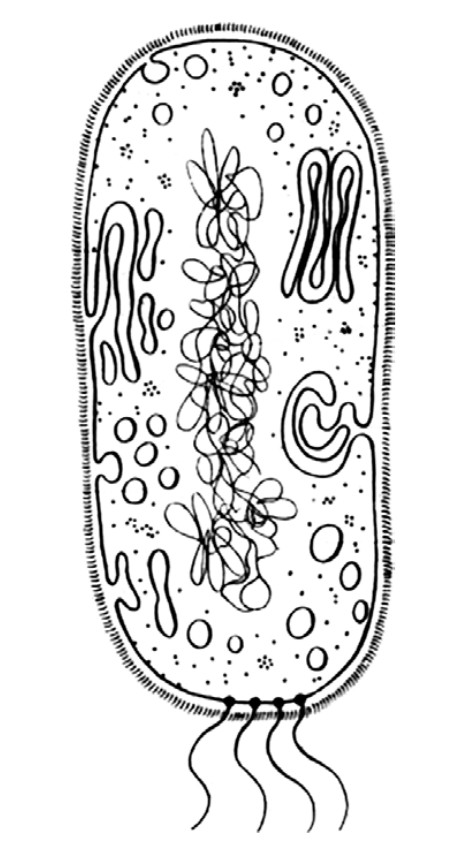
Fig. 1.29. Polycentric structure of a prokaryotic bacterial cell (no spherical shape, nucleus and shells)

They have a nucleus, which contains all the genetic material and controls their development. This is the first thing that distinguishes them structurally from prokaryotic cells (see Fig. 1.29), although, of course, they have many other differences85. In prokaryotes, the genetic material is located in the cytoplasm and is not protected by anything. There is no true nucleus and no nucleolus. In eukaryotes, the genetic material is located in chromosomes inside the nuclei.

What is important for us in this case is that prokaryotes are mainly 0.5 to 5 microns in size, while eukaryotes are usually up to 40 microns. Consequently, the nucleus - as a sign of monocentricity - appears in cells (eukaryotes) whose size exceeds 10 µm.

This does not mean that cells with a nucleus are never less than 10 µm, but most of them are still in the size range of 10 to 100 µm.

Obviously, the cell nucleus, the size of which is on average close to 10-30 µm, is a striking sign of M-structure for living systems. However, there are practically no other signs of monocentricity in them. All cells (eukaryotes) by definition have at least one sign of monocentricity - the nucleus (see Fig. 1.28), but their shape is mostly non-spherical. However, the absence of spherical shape of a cell may be a consequence of the fact that neighboring cells closely adjoin it, and they distort the natural potentially spherical shape of a single cell of a multicellular organism. In fact, many simplest organisms have a much more centrosymmetric structure - cells that exist autonomously, such as radiolaria (see Fig. 1.30), their sizes lie mainly in the range of 40-100 microns. It is not for nothing that they got their name; their radius-radius structure is the 4th additional sign of monocentricity. In addition, many of them have a spherical shape, a central core and sometimes shells.

"A characteristic feature of the structure of radiolarians is the presence of a central capsule ... In some species are formed not one, but several balls, nested in each other and connected by radial needles."

Animal life. Invertebrates. Т. 1 // Edited by L. A. Zenkevich. - Moscow: Enlightenment, 1968. С. 88, 90.

After the size of 100 µm, monocentricity practically recedes (see Fig. 1.27B). Even radiolarians with larger sizes can take an already not so spherical shape or completely non-spherical and even polycentric (see Fig. 1.31). For unicellular organisms with sizes greater than 100 µm (e.g., infusoria), only one of the three main features of monocentricity is inherent in the presence of a nucleus.

Fig. 1.30. Radiolarians with sizes up to 100 µm possess radial-radial symmetry and have a central nucleus. The figure shows the "skeletons" of these radiolaria

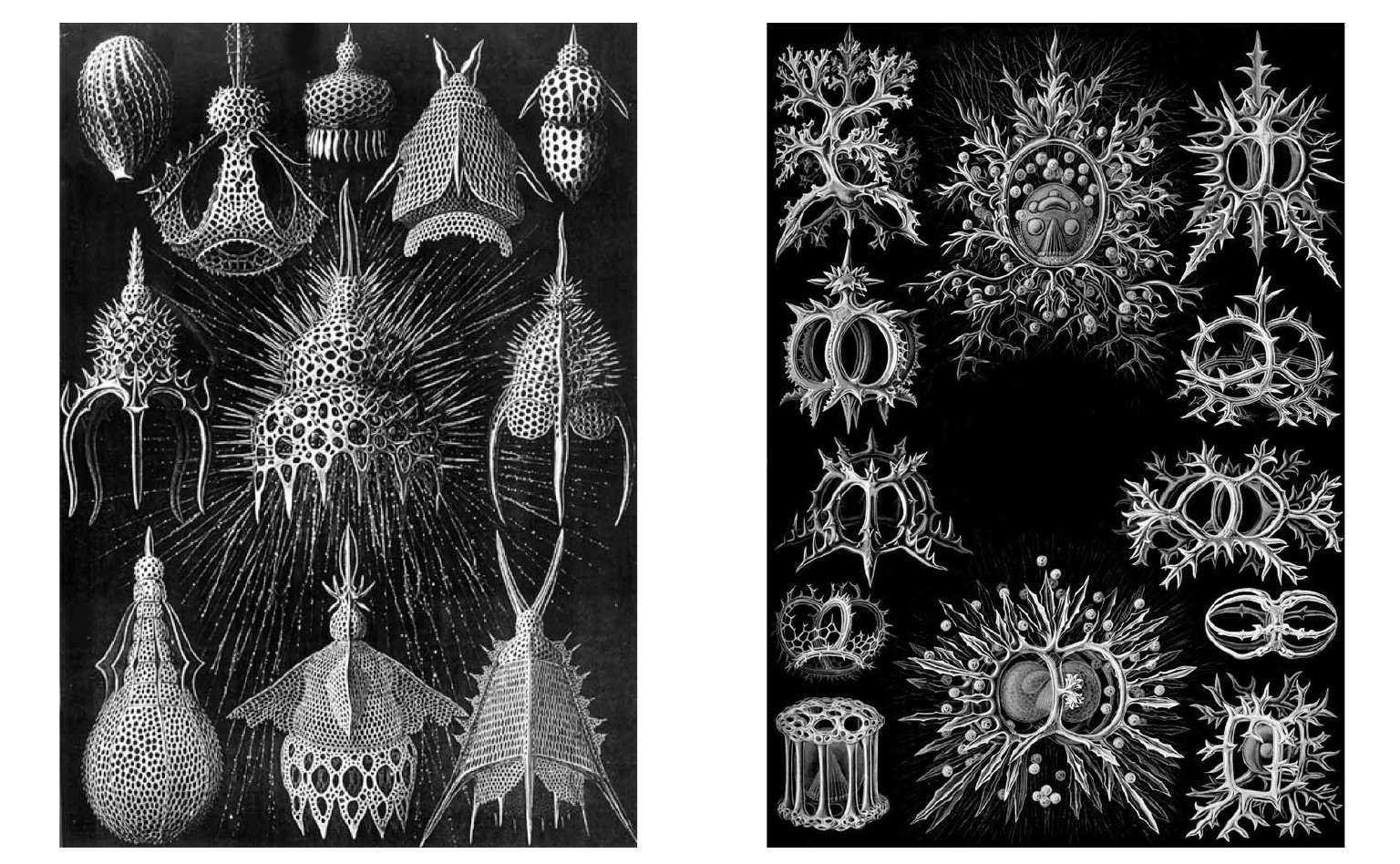Fig. 1.31. Radiolarias with sizes greater than 100 µm generally have significantly weakened signs of central-radial symmetry compared to smaller individuals

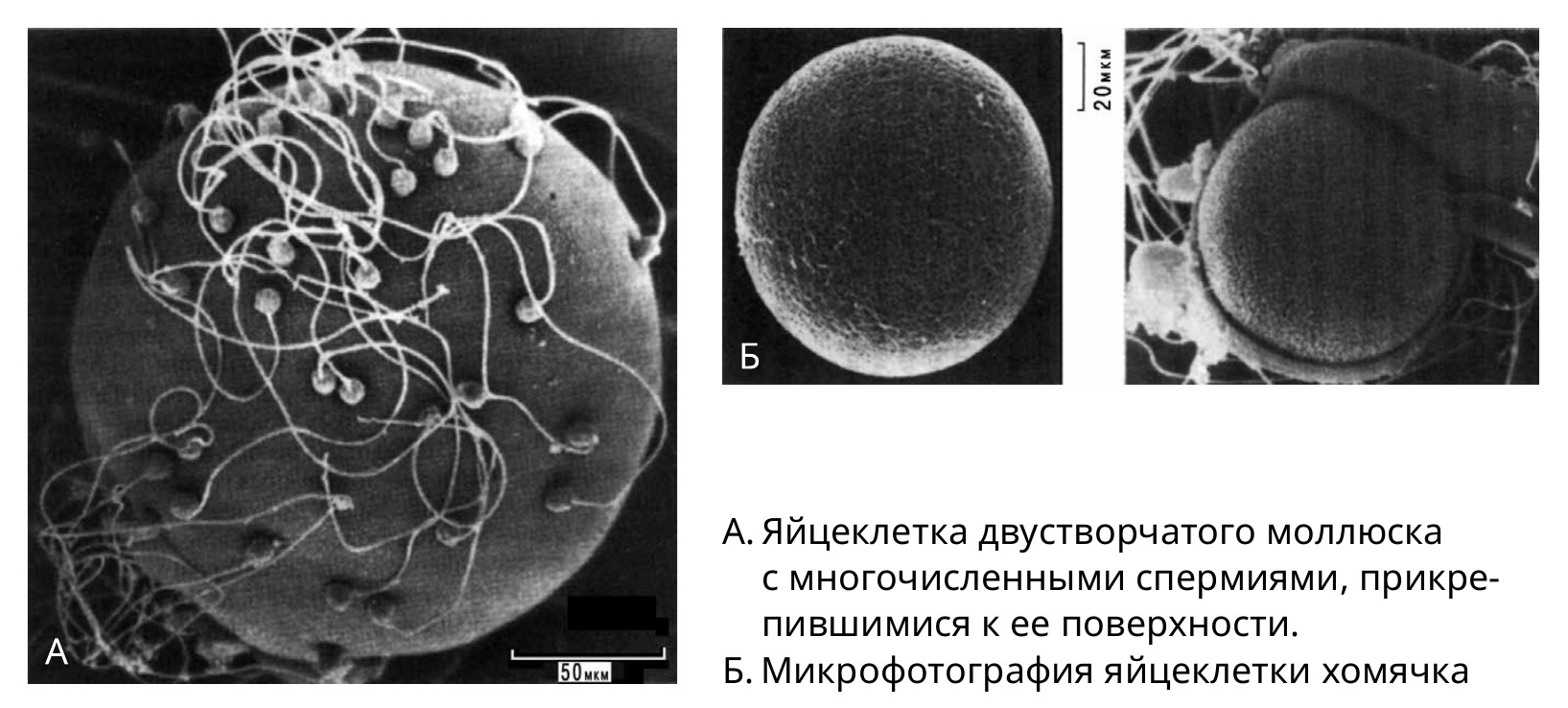Fig. 1.32. Sexual spherical cells

Thus, we can confidently assert that for protein systems in motion along the S-axis, monocentrism is manifested mainly in the size range of 10-100 µm.

Most pronounced in the germ cells of any organisms, and, in addition, in radiolarians of corresponding size.

Movement along the S-axis beyond 100 µm leads to the dominance of P-type structures and almost complete disappearance of M-type structures (see Fig. 1.27B). The exception is large germ cells, which are most often spherical in shape (see Fig. 1.32) and in some cases reach several centimeters in size (bird eggs, for example).

Therefore, it may be noted that beyond the 100 µm limit, sphericity appears primarily where the system is functionally closest to the reproduction process.

This phenomenon can be called a large-scale memory of shape: germ cells, apparently, at the very beginning of the evolution of biosystems always had sizes from 10 to 100 μm, and only later some of them acquired larger sizes, but they retained sphericity and other signs of monocentricity.

This memory of form is reflected in the eggs of birds and in the seeds of many plants. But what is interesting: signs of monocentricity are manifested as shape memory most strongly in the reproductive organs of many multicellular organisms. For example, ovaries in animals are the organs closest to spherical symmetry, and flowers in plants - to radial symmetry.

If we do not take these and other rare exceptions (sea urchins and similar spherical and radial-radial forms of animals and plants), it is obvious that after the transition from cells to cellular organisms, monocentrism disappears and is replaced by polycentrism again.

This is very clearly manifested in the process of development of the germ cell, which immediately after the first division, turning into a blastula (see Fig. 1.33), goes from a monocentric form to a polycentric - multinucleated, although still mainly spherical.

Thereafter, polycentrism dominates at all scale levels, up to the transition through point B on the S-axis.

In parallel with the loss of monocentric features, as one moves from the 10-100 μm range toward larger systems, the symmetry elements and the degree of symmetry are rapidly lost. As is known86 , the highest symmetry is possessed by a sphere with a center of symmetry, and the lowest symmetry group is mirror symmetry. Therefore, starting from radiolarians and germ cells, which have symmetry close to the maximum, the transition to larger and larger bioorganisms is accompanied by the loss of symmetry, until for many animals, out of all kinds of possible symmetries in Nature, only one - mirror symmetry, the lowest type of symmetry - remains.

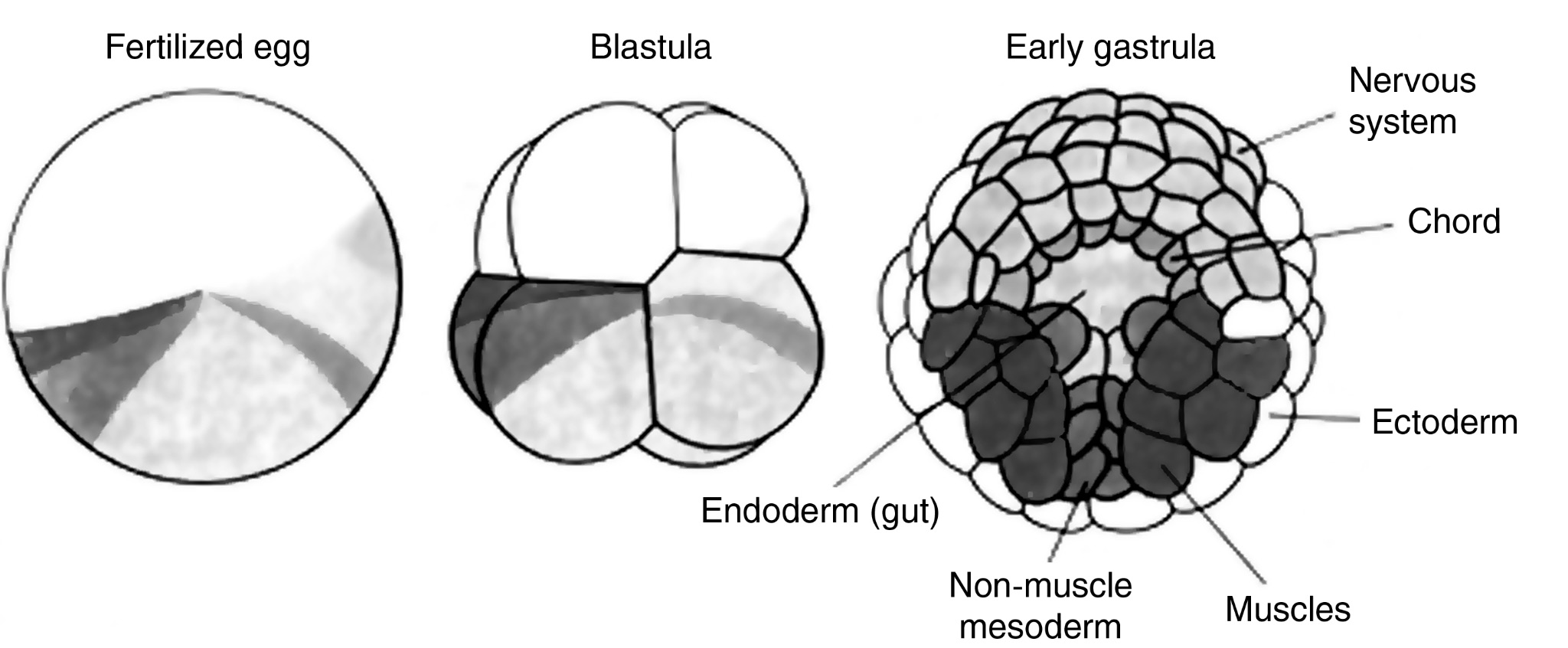Fig. 1.33. Departure from central symmetry in biosystems. Three successive stages of an organism's early development are shown. It is evident how nature is rapidly moving away from monocentrism to polycentrism.

Beyond the \(10^{2}\) cm SWS ridge (on which humans "sit"), social and biological community systems mostly begin, as rare animals cross the meter range and rare plants cross the ten-meter range. Polycentric structures also dominate here. Moreover, the symmetry of form and structure is almost completely lost here. So, let us finalize the consideration of biosystems and return to the consideration of the MACRO-INTERVAL as a whole. Let us summarize the results.

Throughout the entire Macro-interval, regardless of the type of systems and their origin, one pattern can be traced: the first order is occupied by transitional forms, the next four orders are exclusively monocentric forms, the remaining 15 orders are mainly polycentric forms.

This allows us to schematically depict the Macro-interval as a two-hump curve (see Fig. 1.26) with a length of 20 orders of magnitude, where the first hump and its top are "populated" by monocentric structures, and the rest of the space - by polycentric structures of different types.

The only exception in the polycentric section is the depression between the humps (SCU), where one order is populated by monocentric structures, although with not fully expressed features. Moreover, despite the huge difference in structure and formation conditions between cosmic and volcanic dust, unicellular organisms and cells within organisms, in the range 10-100 μm, which exactly corresponds to the middle part of the macrowave (and the bottom part of the highest stability at the corresponding section of the HU), there is a noticeable and very statistically significant increase in symmetry, which is manifested mainly in the radial-spherical shape and the presence of a nucleus.

These facts allow us to assume the existence of a SINGLE MASSIVE FIELD WHICH REGULATES THE DEGREEOF SIMMETRY AT ALL LEVELS OF THE HIERARCHY OF THE UNIVERSE, about which we will speak further.

Summarizing all the above, it can be noted that electromagnetic forces immediately after the beginning of the Macro-interval manifest themselves in the structural arrangement of atoms very brightly. Here, as if their qualitative formation takes place. At the remaining 15 orders, there is a quantitative increase of both the mass of structures and their complexity.

  1. Zafiratos K. D. Structure of the nucleus surface // Physics of the atomic nucleus and plasma. M.: Nauka, 1974. P. 45. ↩

  2. Baranger M., Sorensen R. A. Size and shape of atomic nuclei // Physics of atomic nucleus and plasma. M.: Nauka, 1974. P. 30. ↩ ↩2

  3. Zafiratos K. D. Structure of the nucleus surface // Physics of the atomic nucleus and plasma. M.: Nauka, 1974. P. 28-43. ↩ ↩2

  4. Baranger M., Sorensen R. A. Size and shape of atomic nuclei // Physics of atomic nucleus and plasma. M.: Nauka, 1974. P. 37. ↩

  5. Zafiratos K. D. Structure of the nucleus surface // Physics of the atomic nucleus and plasma. M.: Nauka, 1974. P. 44. ↩ ↩2

  6. Mukhin K. N. Physics of atomic nucleus // Experimental nuclear physics. Т. 2. M.: Atomizdat, 1974. P. 273. ↩

  7. Mukhin K. N. Physics of atomic nucleus // Experimental nuclear physics. Т. 2. M.: Atomizdat, 1974. P. 277. ↩

  8. Ginzburg V. L. On Prospects of Development of Physics and Astrophysics at the End of the Twentieth Century // Physics of the Twentieth Century. Development and Prospects. Moscow: Nauka, 1984. P. 281-330 ↩

  9. Zheludev I. С. Symmetry and its applications. Moscow: Atomizdat, 1976. P. 14. ↩